The Mark Ortiz Automotive

CHASSIS NEWSLETTER

Presented free of charge as a service

to the Motorsports Community

September –November 2004

WELCOME

Mark Ortiz Automotive is a chassis consulting service primarily serving oval track and road racers. This newsletter is a free service intended to benefit racers and enthusiasts by offering useful insights into chassis engineering and answers to questions. Readers may mail questions to: 155 Wankel Dr., Kannapolis, NC 28083-8200; submit questions by phone at 704-933-8876; or submit questions by

e-mail to: markortiz@vnet.net. Readers are invited to subscribe to this newsletter by e-mail. Just

e-mail me and request to be added to the list.

ERRATA AND ADDENDA

Thank you to readers who have pointed out some detail discrepancies in my recent columns in Racecar Engineering, which are drawn from this newsletter.

In the April newsletter I originally stated that for least bearing loads with an outboard brake, the caliper should be somewhere in the upper rear quadrant of the disc. Shortly after sending out that issue, I sent out a correction saying that should be the lower rear quadrant. This correction was supposed to be incorporated when the material was published in the magazine, but unfortunately the original version was what ran (July 2004 issue). So a correction is in order on this point, for those who read me in the magazine.

In my October 2004 column, drawn from the June 2004 newsletter, there was some disagreement between what I said about the Williams Formula 1 suspension and the second picture that ran in the magazine, showing the suspension from above and behind. I had said the forward portion of the upper wishbone was not aligned with the tie rod, whereas in the picture it appears to be aligned. Some readers have understandably called this to my attention.

The picture in question was chosen by the magazine, not supplied by me. I based my comments on other pictures, which I did not have in electronic format. If anybody is to be faulted here, I am. Anyway, it appears there have been two versions of the suspension. In the version shown in the magazine, the bump steer and aerodynamics appear good, but the camber control properties appear poor. In the version I was looking at when I wrote the text, the control arm appears to have been leveled out by moving the forward pickup point down a bit, without the steering rack being lowered to match.

Without inside knowledge of the team’s internal affairs, I am of course speculating as best I can from partial evidence. The best explanatory theory I can devise is this: the original version had the wishbone and the tie rod as shown in the October column’s illustration. Perhaps the camber control was consciously compromised to get the nose higher and aid airflow underneath it, which was also partially the object of the tusk nose design. To get the floor of the nose up, the driver’s feet had to go

up. That forced the steering rack, the pedals, and everything else to go up. The wishbone then had to agree with the steering, to prevent bump steer and get good airflow over the tie rod and wishbone.

When it was found that the car lacked front grip, and other fixes didn’t cure the problem, the team tried moving the wishbone pickups down. Moving them down a little on the existing tub was feasible, but moving the steering rack was tougher. So as a temporary experiment and hopefully a temporary solution, the team decided to accept some bump steer and rely on driver skill to deal with that, and see if improved camber control would help the grip.

Raising the upper ball joints and outer tie rod ends was not an option, because the wheel rim was in the way. Lowering the rack was not an option, because the driver’s feet (or maybe other elements of the car) were in the way. So the team made the only modification they could, under the circumstances.

I also noticed elsewhere in the October issue that as of the Hungarian Grand Prix the team had abandoned the tusk nose design, although the tub has not been re-done.

I understand they are going to try an entirely new approach for 2005.

TIRE LOAD SENSITIVITY AND WEIGHT TRANSFER IN TRAILBRAKING

In the Forum (letters) column of that same October issue was a letter from a physicist, responding to an earlier article on the theory of cornering line and trailbraking by Erik Zapletal. Erik had correctly noted that forward load transfer (weight transfer, in customary vernacular) tends to improve the lateral acceleration capability of the front wheels, at the expense of the rear ones. The physicist took issue with this, and pointed out that adding weight to a wheel pair reduces lateral acceleration capability, because due to the phenomenon we call tire load sensitivity, the coefficient of friction diminishes as we add load. Mr. Zapletal replied that this is true, but load sensitivity is a minor effect. (He also noted that other factors enter into this, including aerodynamics, brake bias, and camber changes.)

Who is correct? Both are, partly. But I think I can explain the matter a little better.

By the way, Erik is a sharp guy and it was he who first pointed out my aforementioned error on brake caliper location. Hopefully, I am returning a favor and shedding some light here, not being a pain.

This question illustrates perfectly why I prefer to speak of load transfer rather than weight transfer. The effects of forward load transfer under braking are quite distinct from the effect we get if we move mass forward in the car. Moving mass forward in the car adds understeer. Forward load transfer in braking adds oversteer. Both effects can be said to relate to tire load sensitivity.

When we move mass forward in the car, we increase both the normal (vertical or perpendicular to the road) force on the front tires and the centrifugal (inertial) force the tires must overcome to produce any given lateral acceleration. Consequently, the front end’s lateral acceleration capability depends not on whether the force capability of the tires increases, but on whether it increases at the same rate as the normal (and centrifugal) force. The ratio of the tire’s force capability to the normal force is the coefficient of friction. This diminishes with increasing normal force, so in any situation where we add weight to the front end, the lateral acceleration capability diminishes.

When we brake, however, most of the load increase on the front wheels does not come from mass moving forward on the wheelbase, although a small amount of such motion does usually occur. The increase in front wheel loading comes primarily from the forward pitch couple which inevitably results from the tires exerting a rearward force at ground level and the car’s inertia exerting an equal and opposite forward force above ground level. To prevent the car from somersaulting, the front tires exert an increased support force against the ground, and there is a corresponding decrease in support force at the rear, creating an equal and opposite anti-pitch couple. Because the center of mass has not moved appreciably on the wheelbase, the front wheels are not required to overcome an increased centrifugal force per unit of centripetal acceleration in proportion to their increased normal force. The normal force increases, while centrifugal inertia force for a given car-lateral (centripetal) acceleration remains largely unchanged. Consequently, lateral acceleration capability for the front wheel pair increases.

In both cases, the normal force increases and the coefficient of friction decreases. But in the former case, the centrifugal force per unit of acceleration increases with the normal force, whereas in the latter case it does not.

The latter case may be said to be similar to what happens when we add aerodynamic downforce. We add significant normal force, or load, without adding significant mass.

WHY ARE WIDE TIRES BETTER?

It has been recognized for about 40 years now that wide tires provide more grip, at least when we are not limited by aquaplaning. One might suppose that this effect would be be well understood by now, on a theoretical level as well as a practical one. Yet the matter seems to be receiving a lot of attention from various authors lately. This seems to be due in part to the need for mathematical tire models to be used in computer simulation. I have encountered the question at least twice in the past month, once in a seminar presented by Paul Haney, based on his recent book about tires, and once in Paul Van Valkenburgh’s November Racecar Engineering column. The issue has also come up in my work as an advisor to the UNC Charlotte Formula SAE team.

On the face of it, one might wonder why there is any controversy about this, and also why it took people until the 1960’s to try wide tires. More tire, more rubber on the road. More rubber on the road, more traction – right? Why wouldn’t this be obvious?

Essentially, there are two reasons it wasn’t obvious. First, according to Coulomb’s law for dry sliding friction, friction is independent of apparent contact area. It depends instead on the nature of the substances in contact, the normal (perpendicular) force, and nothing else. Second, a tire’s contact patch area theoretically doesn’t vary with its width anyway. If we widen the tread, the contact patch just gets shorter, and the area theoretically stays the same.

Let’s consider each of these notions. Coulomb’s law applies quite accurately to hard, dry, clean, smooth surfaces. However, a tire tread is a soft, tough, sometimes tacky substance in contact with a hard, rough surface. When two hard, smooth surfaces are in contact, they actually touch only at a small percentage of their apparent or macroscopic contact area. Friction depends on molecular bonding in the small microscopic contact zones. As normal force increases, the microscopic contact area increases approximately proportionally, and consequently friction is directly proportional to normal force.

With rubber on pavement, however, there is not only the usual molecular bonding but also mechanical interlock between the asperities (high points) of the pavement and the compliant rubber. Sliding then involves a combination of shearing the rubber apart and dragging the asperities through it as the rubber reluctantly oozes around the asperities. The interface somewhat resembles a pair of meshing gears. With gears, when we increase the size and number of teeth in mesh, we increase the force required to shear off the teeth. It would be reasonable to expect a similar effect with the interlock between the tread and the pavement.

With increasing normal force, this interlock gets deeper, as the asperities are pushed further into the rubber. However, we might reasonably expect that at least beyond a certain point, the asperities are pushed into the rubber to pretty nearly their full depth, and further increase in normal force does not proportionately increase the mechanical interlock. With greater macroscopic contact area, it should take a greater normal force to reach this region of diminishing return.

A tire typically does show characteristics that would match this hypothesis. It will often have a range of loadings where its coefficient of friction is almost constant; where friction force is almost directly proportional to normal force. Above this range, the tire exhibits much greater load sensitivity of the coefficient of friction. The curve of friction force as a function of normal force goes up almost as a straight line for a ways, then begins to droop at an increasing rate.

Of course, the contact patch does not remain the same macroscopic size as load increases. It grows as we add load. Nevertheless, this contact patch growth is evidently not enough to keep the coefficient of friction constant.

The contact patch growth is interesting in itself, and a bit counter-intuitive. A tire can be considered a flexible bladder, inflated to some known pressure, and supporting a load. If such a bladder is extremely limp when uninflated, like a toy balloon, and we inflate it, place it on a smooth, flat surface, and press down on it with a known force, the area of contact with the surface is equal to the normal force divided by the pressure:  A = F_n/P.

If a tire approximates this behavior, then it follows that the contact patch area depends only on the

load or normal force and the inflation pressure. If we make the tire wider, then at any given load and  pressure the contact patch doesn’t get bigger, it just gets wider and shorter.

Accordingly, much discussion of the reasons a wide tire gives an advantage focuses on reasons we might expect a wider tire to yield greater lateral force than a narrower one, assuming similar construction and identical pressure, tread compound, and load.

One theory, advanced by the late Chuck Hallum and evidently picked up by Paul Van Valkenburgh in his recent column, is that a tire is primarily limited by thermodynamics. It generates drag when running at a slip angle. The drag times the speed equals a power consumption, or rate of energy flow. This energy is converted into heat. For the system to be in equilibrium, the heat must be dissipated as fast as it is generated. Even short of the point of true equilibrium, the tread compound needs to be kept below a temperature where it softens to the point of being greasy rather than tacky. If the contact patch is shorter, that means that each square inch of tread surface spends less time getting heated and more time getting cooled.

Also, when a tire is operating near its lateral force limit, the front portion of the contact patch is “stuck” to the road and the rear portion is a “slip zone” in which the tread moves across the pavement in a series of slip-and-grip cycles. The slip zone grows as we approach the point of breakaway. Beyond the point of breakaway, the entire contact patch is slip zone. The slip zone generates less force and more heat than the adhering zone. A shorter, wider contact patch is thought to have a larger adhering zone and a smaller slip zone at a given slip angle, and wider tires are also known to reach peak force at smaller slip angles. Therefore, a wider tire is not only better able to manage heat, but also generates less heat at a given lateral force.

This all makes sense, but it fails to explain why wide tires give more grip even when stone cold.

There is little doubt that they do. If you have a street car with four identical tires, and you replace the rear tires and wheels with ones an inch wider, using the same make and model of tire, with no other changes, the handling balance will shift markedly toward understeer. You will see this effect at all times, from the first turn in a journey to the last. Surely this effect is not coming from heat management.

Paul Haney explains this by the larger-adhering-zone theory described above. The tire makes more efficient use of its contact patch, even if the contact patch isn’t larger.

As much sense as the above theories make, they ignore some real-world effects that have a bearing on the situation.

First of all, the degree to which tires follow the A = F_n/P rule varies considerably. A very flexible tire, at moderate load, may have a contact patch as large as 97% of theoretical. A fairly stiff tire may

be well below 80%. We are all aware of run-flat tires currently being sold, which will hold up a Corvette with no inflation pressure at all. As P approaches zero, F_n/P approaches infinity. If A does

not approach infinity, and the tire does not go flat, the contact patch area as a percentage of theoretically predicted area approaches zero.

One might suppose that the effect of carcass stiffness would be significant mainly in street tires, with run-flats being an unrepresentative extreme. Yet I have seen dramatic differences in carcass rigidity in different makes of racing tires intended for the same application. The Formula SAE car run by the University of North Carolina Charlotte uses 10” wheels. Hoosier and Goodyear both make 6” nominal-width tires for the application. The stiffnesses of these tires differ dramatically. The Hoosiers are much more flexible than the Goodyears. The Goodyears are so stiff that they will support the front of the car (without driver), with little visible deflection, when completely deflated – run-flat racing tires! How closely do these tires approximate A = F_n/P in this load range? Not very closely at all.

My point here is that tire stiffness, vertically, laterally, and otherwise, is not purely a function of inflation pressure, so it is a bit risky to try to infer contact patch size from pressure and load. Therefore, we don’t necessarily know that two tires differing only in width do have the same contact patch area at the same inflation pressure and load, or even that tires of the same size do.

Anyway, if it is approximately true that A = F_n/P, it follows that a wide tire will have greater vertical stiffness, or tire spring rate, than a narrow one, at any given inflation pressure. It will also have a smaller static deflection at a given load, which is why the contact patch is shorter. The flip side of this is that for a given static deflection or tire spring rate, a wide tire needs a lower inflation pressure. Consequently, if we compare wide and narrow tires at similar static deflection or tire spring rate, rather than similar pressure, they will have similar-length contact patches and the wider one really will have more rubber on the road, just as we would intuitively suppose from looking at them.

As we make a tire wider, not only does vertical stiffness increase for a given inflation pressure, so does the tension in the carcass due to inflation pressure. A tire is a form of pressure vessel. We may think of it as a roughly cylindrical tank, bent into a circle to form a donut or torus. Borrowing from the terminology of pressure vessel design, we may speak of the “hoop stress” in the walls: the tensile stress analogous to the load on a barrel hoop. For a given inflation pressure, the hoop stress is directly proportional to the cross-sectional circumference, or mean cross-sectional diameter. When the carcass is under a higher preload, the tire acts stiffer laterally. This effect can easily be seen in bicycle tires. A fat bicycle tire will feel harder to the thumb than a skinny one, at any given pressure. If we try to inflate a mountain bike tire to the pressure we’d use in a narrow road racing tire, the tire will expand its bead off the rim and blow out. So when we compare narrow and wide tires at equal inflation pressures, the wider one will be stiffer laterally as well as vertically, and it will achieve this at no penalty in contact patch size.

Finally, there is the question of tread wear. As we have noted, if the contact patch is longer, it has a larger slipping zone near the limit of adhesion, and it also spends a greater portion of each revolution

in contact with the road. Not only do these factors influence how hot the tire runs, they also influence how fast it wears. Therefore, assuming good camber control, a wide tire should last longer than a

narrow one, with similar tread compound. The astute reader will see where I’m headed with this. If we need to run a given number of laps or miles on a set of tires, then with wider tires we can trade away some of the inherent longevity advantage, and run a softer compound.

Okay, summing up, what does a wider tire get us?

1. It runs cooler, and/or

2. it makes more efficient use of its contact patch by having a greater percentage adhering, and/or

3. it can run at lower inflation pressure and therefore actually have a larger contact patch, and/or

4. it can have greater lateral stiffness at a given pressure and therefore keep its tread planted better, and/or

5. it can use a softer, stickier, faster-wearing compound without penalty in longevity.

Note that most of these effects in turn play off against each other. We can blend and balance them, and get a tire that is somewhat cooler-running, has a somewhat lower operating pressure and somewhat larger contact patch, has somewhat greater lateral stiffness, and survives long enough with a somewhat stickier compound, all at the same time. That would explain an improvement in grip, wouldn’t it?

REAR PERCENTAGE VERSUS YAW INERTIA

Setting up race cars is invariably a compromise.  Most of these are well documented, but I have found little on the compromise between longitudinal weight distribution and polar moment of inertia.

I race a 250bhp, 900K V8 MGB which, unfortunately for a rear-wheel-drive car, has a frontal weight bias of around 60%.  The fuel tank of around 40L overhangs the rear axle, which creates a moment around the rear axle and thus helps to remove weight from the front.

There is space in front of the rear axle to place two tanks where the batteries would have gone on the road car.  This would have the effect of reducing the polar moment of inertia while slightly lowering the center of gravity and possibly allowing softer rear springing.  It would also improve safety (as long as it is protected from the prop shaft running between the two tanks!).  However, it would increase the front bias.

This would be quite a lot of work and expense, so I would be grateful if you could comment on the benefits or otherwise of this approach.

From a vehicle dynamics standpoint, I would opt for more rear percentage rather than less yaw inertia, especially in a car that is so nose-heavy now.

Within limits, yaw inertia can be coped with by driving technique. In some situations, it can even make the car faster. Overall, though, less yaw inertia is better, particularly when the course demands high yaw accelerations, as when negotiating chicanes or street-circuit turns that come in quick succession. But steady-state handling and ability to put power down are more important. A rear-drive car with only 40% rear weight and a powerful engine is seriously traction-challenged, especially when exiting turns. Any further reduction would not be good.

With more front percentage, you will actually have to stiffen the rear suspension, at least in roll, with respect to the front. Otherwise, you will be adding understeer. The inside rear will then be extremely light when cornering. It probably is now.

One situation where yaw inertia can make a car faster is where the car may unexpectedly encounter a slippery spot in the middle of a turn. If the slippery patch is short enough so the front and rear of the car hit it separately, the car will experience understeer and then oversteer in very quick succession: it will do a wiggle. If it has little yaw inertia, it will do a big wiggle. If it is close to the limit, it may spin. If the driver wants to allow a margin of safety to increase the chances of catching the wiggle before it becomes a spin, for a given level of risk the driver must stay further from the limit in a car with little yaw inertia.

For this reason, in the days of high-speed open-road racing, many engineers regarded yaw inertia as desirable, and this was thought to be one of the advantages of a front-engined car with a transaxle, and a problem for the rear-mid-engine layout.

Even back then, everybody recognized that weight distribution change with fuel burn-off was not good. The Lancia-Ferrari of the mid-1950’s, with its pontoon fuel tanks between the front and rear wheel on each side, appears designed to get much of the fuel amidships longitudinally, while preserving high yaw inertia. As Ferrari developed the car after taking it over from Lancia, they moved the side tanks inside the body, reducing yaw inertia, and this is generally thought to have improved the handling.

It is worth noting that the Lancia-Ferrari predated foam-baffled fuel containers. Even with some sheet metal baffles, there must have been considerable fuel slosh in those tanks, and that can’t have been good for controllability.

As regards crash safety and fire risk with the fuel inside the wheelbase versus outside, there are pros and cons both ways. If the fuel is within the wheelbase, it is less likely to spill when the rear takes a hit. On the other hand, if it does spill, it is more likely to spill into the driver’s compartment. And it can still spill, as recently demonstrated in Dale Earnhardt Jr.’s crash in the Corvette at Sebring.

At the recent SAE Motorsports Conference in Dearborn, Michigan, I had the opportunity to ask a very distinguished panel of safety experts about the safety aspects of fuel location. Gary Nelson of NASCAR said that they strongly considered having the fuel ahead of the axle in the new NASCAR

chassis they are developing, but decided against it. The reason, he said, was that the greatest risk of

fuel fires occurs in refueling during pit stops. The present rear location was considered preferable from that standpoint. This may be less of a factor where the races are short and there are no fuel stops.

ROLL SPRINGING PRELOAD IN MONOSHOCK SETUPS

I race a 1998 Dallara F398 F3 car in an amateur series in the UK.  The car is fitted with a monoshock front suspension with Belleville washer stacks to control lateral movement of the rocker. I have attached a couple of photos and a copy of the setup page from the Dallara build manual.

[To keep file size manageable for e-mailing, I am including only one photo in the newsletter. As it appears here, it’s on its side. Rear of the car is to the left, left side of the car is up. The assembly shown is located on top of the footwell area of the monocoque tub.]

My questions concern spring preload.  I have read many books and can find little information on the subject.

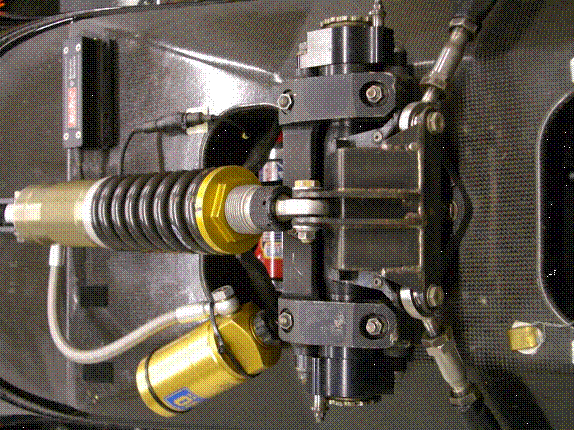
Question 1:  The front spring is preloaded so that in a static condition there is sufficient load to support the weight of the car, i.e. there is no front droop travel.  Can you explain to me the effect of increasing the amount of preload compared to, say, fitting a stiffer spring with less preload?  Does this cause the car to behave differently in low-speed corners when there is little downforce, compared to high-speed corners?

Since the coilover only acts in ride, its action only affects the wheel rate in ride, and has no effect on the wheel rate in roll. It does affect ride height, and that has effects on both downforce (higher ride height makes less downforce, as a rule) and geometric roll resistance (roll center height – higher front ride height means more front roll resistance, as a rule, because the roll center usually rises and falls with the sprung mass).

With a setup as the questioner describes, with preload just equal to static load, if there is no downforce, the wheel pair cannot extend synchronously (in ride) past static position, but one wheel of the pair can if the other moves into compression a similar amount. If there is slight downforce, as at low speed, there can be a little ride movement in the extension direction. When there is a lot of downforce, the suspension can move quite a bit in ride extension before it reaches static position and tops out.

What happens if we preload the suspension more in ride? Assuming non-spring components to be rigid, we then get no ride motion at all in either direction, until force in the compression direction (from aero, road irregularities, or rearward acceleration, or any combination of these) overcomes the preload.

If, on the other hand, we add spring rate and not preload, we get less ride motion in compression for a given load increase, but we always get some.

So a stiffer spring begins to move with any load increase. A softer spring, with more preload, doesn’t yield at all until the preload is overcome, but then moves more per unit of load increase.

Well, do we want the suspension to move more or not? It’s a tradeoff. If the suspension moves more, wheel loads change less, but camber, suspension geometry, and aerodynamic properties change more. If we cinch down the suspension so it can’t move, then we keep camber and geometry from changing, but wheel loads will vary greatly over irregularities, and the wheels may even become airborne. We get good aerodynamic consistency, up to the point where the wheels come off the ground. Beyond that point, ride height and pitch angle can change very rapidly.

Suppose we have to keep the car at a certain height at a certain peak speed, with a certain aero package. Suppose we have a choice of a soft ride spring, heavily preloaded, or a stiff ride spring, set to zero droop but with no other preload, or the stiffer spring allowed to move in droop.

What would give better behavior in low-speed corners? If there are bumps to contend with, the stiff spring not preloaded will be best, because it will alow the wheels to follow the bumps best. The stiff

spring set for zero droop will be next best. The soft spring heavily preloaded will be worst, because it will essentially be locked solid in both directions.

If, on the other hand, the track is very smooth, the heavily preloaded ride spring may actually confer a slight advantage, because it will control camber a little better due to the lack of ride motion.

These are the same basic advantages and disadvantages we see with soft and stiff setups generally.

Question 2:  Roll control of the front suspension is achieved by using Belleville washer stacks, which can be configured to various spring rates.  The build manual recommends minimum and maximum amounts of preload that should be applied to each stack configuration.  I understand that if the preload is lost on one of the stacks during roll, then the total stack stiffness suddenly halves, which is why there is a minimum preload.  I guess that the maximum value is ther to ensure that stacks do not go solid.  Apart from the above, am I right in thinking that changing the Belleville preload will have no effect on the car?

Assuming the washers themselves are fairly linear, as suggested by the listing of a single rate for each stack configuration, there should be no change in handling properties or rates with variations in preload, provided nothing unloads or goes solid.

You might be able to test the washers to see how linear they really are.

It will be apparent that you could create stacks that are non-linear if you wanted to. To do that, you would have to have some of the washers doubled, tripled, or quadrupled, and others not. None of the factory stacks include anything like that.

I wouldn’t really suggest trying deliberately non-linear stacks, but if you did, that would create a situation where the system would become more sensitive to preload. If you had enough preload to compress the softer portions of the stacks to solid when the rocker is centered, you could create a stepped falling rate. If you had the softer portions not compressed to solid with the rocker centered, you could get a stepped rising rate instead.

I imagine you don’t race on ovals, but this could get interesting when using asymmetical setups with diagonal percentages other than 50%.

WHY SO FEW REAR MONOSHOCK SETUPS?

With monoshocks appearing on more designs at the front, is there any reason, other than packaging, that they are never used on the rear of a car?

The Dallara system above is typical in that the roll mode is undamped. I do think it is possible to incorporate a damper in such a mechanism, but it would involve making your own, and the relatively small travel might be a disadvantage.

The Dallara information sheet has a partial sectional view of the rocker mechanism, showing something that looks like a needle valve, which conceivably might be part of a damping mechanism, but the questioner informs me that it’s part of a position sensor, and there is no roll damping.

With a conventional two-coilover suspension, we get damping in both ride and roll.

If a car has no roll damping at either end, it will oscillate in roll and possibly in warp (opposite roll at the front and rear). Really, we’d like to have roll damped at both ends, and have at least the low-speed roll damping adjustable.

But if we are going to damp only one end of the car in roll, it should be the rear. Here’s why: rear roll damping creates an anti-roll moment at the rear when roll velocity is outward, early in the turn. It creates an anti-de-roll (in other words, pro-roll) moment late in the turn when the car’s roll velocity is inward. That loosens the car (adds oversteer) during entry, and tightens it (adds understeer) during exit. This tends to compensate for the tendency of yaw inertia to create understeer during entry and oversteer during exit.

Actually, we don’t always want this, or if we do, we can have too much of a good thing. Driving style, brake bias, driver preference, and other factors enter into this, so really we’d like adjustable roll damping at both ends of the car. But it’s better to have the roll damping loosening entry and tightening exit than vice versa, for the majority of setups, drivers, and situations.